1. A problem
The most fundamental concepts are the very hardest to communicate. Words only give poser-knowledge; even a story doesn’t suffice.
2. Basic plan
Teach the most fundamental concepts (those that repeat throughout the disciplines) through many examples. Don’t assume that kids will connect ‘em — do it openly, and help them achieve the aha! of seeing that they’re all particular instances of one fundamental pattern.
3. What you might see
In science, natural selection being taught through odd example after odd example:
how did a cheetah get its speed?
how did a spider get its web?
how did an onion get its murdery-ness?
In math, a kid entering multiple solution methods into their Deep Practice Book, and slowly understanding that each of those answers, in order to work, has to secretly be the exact same thing.
In economics, a teacher giving counterintuitive examples of how incentives matter that look like they have nothing in common, and prompting kids to figure out what they all have in common.
4. Why?
Brain science blast: it’s impossible to teach a general concept. Every memory is woven in a web of neurons, and every web is specific. The only way to get a general, abstract concept into the brain is to create many different webs and connect them together, so the brain can make a new web of what they all have in common.
5. Egan’s insight
Where do we see this in the human experience?
Throughout history, people have found it easy to understand generalizations of things they see every day:
after seeing umpteen instances of chairs, our brains create an abstraction of what a “chair” is
after seeing umpteen instances of justice, our brains create an abstraction of what “justice” is
How might this build different kinds of understanding?
Abstract knowledge is at the heart of what Egan dubs PHILOSOPHIC (👩🔬) understanding. Cultivating it has been the explicit goal of academics since the philosopher Plato founded his school (“the Academy” after which all others are named).
To achieve these 👩🔬ABSTRACTIONS, we can use any of the other tools. (What do these weird emoji mean?)
6. This might be especially useful for…
Everyone who’s memorized a superficial instance of something, but who’s then struggled to use that information in a different situation.
7. Critical questions
Q: Isn’t this just part of what all teachers do instinctively, all the time?
Absolutely — but by seeing clearly how this works, we can all do it much more powerfully and quickly. To wit: the more important a concept is, the more examples you should give of it, and the more superficially dissimilar they should be.
Q: What ARE the most important general concepts?
The very concepts that we build curriculum around. In math, this includes things like “place value”, “functions”, and even “numbers” themselves. In science, “ecosystems”, “natural selection”, and “energy”. In history: “urbanization”, “rule”, and “rights”.
Q: Could you give an example?
To understand what a “government” is, it’s not enough to learn details of a particular government — you need to learn the basics of as many radically different governments as possible: participatory democracies, strongman dictatorships, Mennonite magazines, feminist anarchist collectives… And then you need to see the commonalities they share. (When you do, you’ll go aha!)
Want to help us all understand this better by picking at this with questions? Go for it — become a subscriber and join in the comments conversation.
8. Physical space
Nothing required here — like some of our other super-general patterns, this already infuses everything.
9. Who else is doing this?
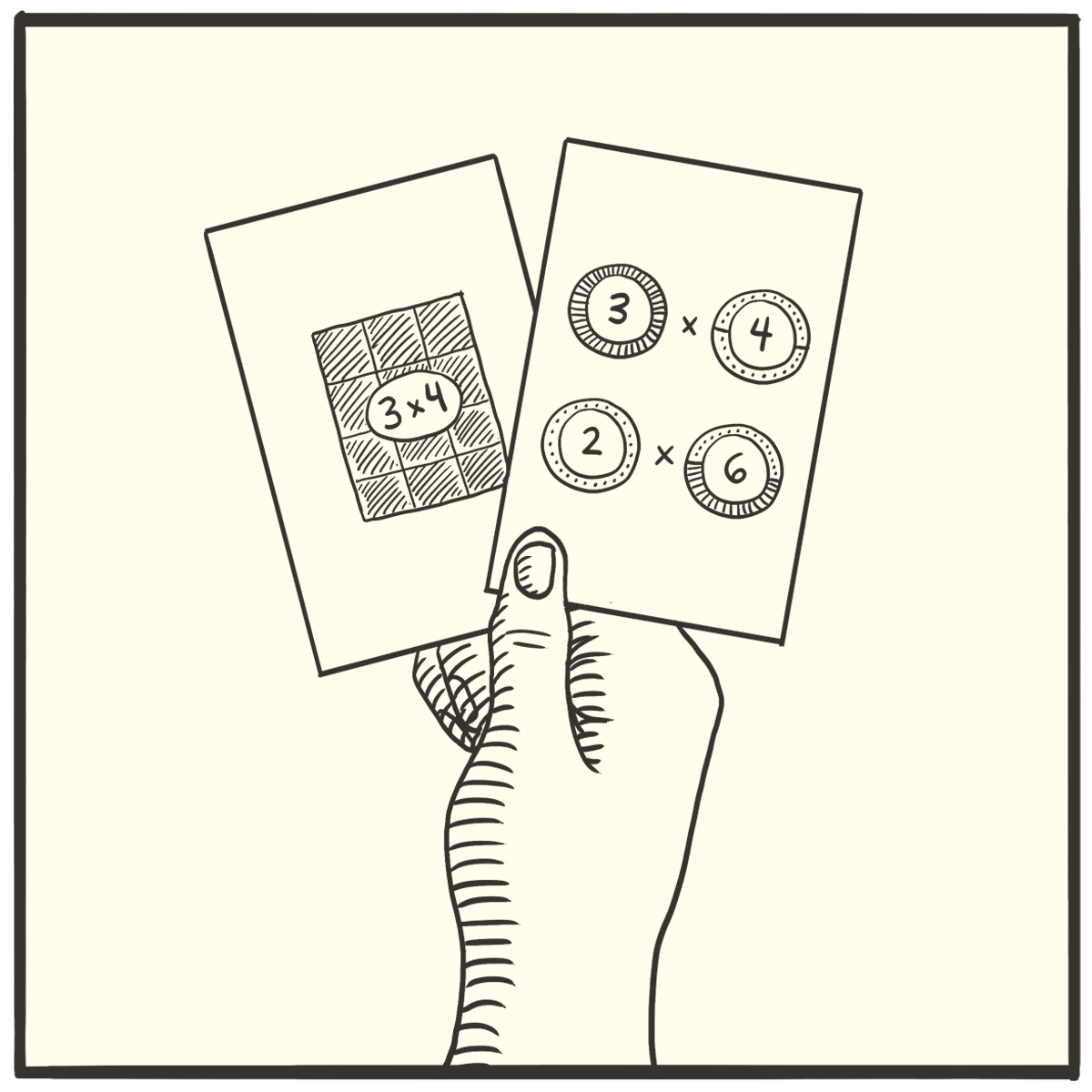
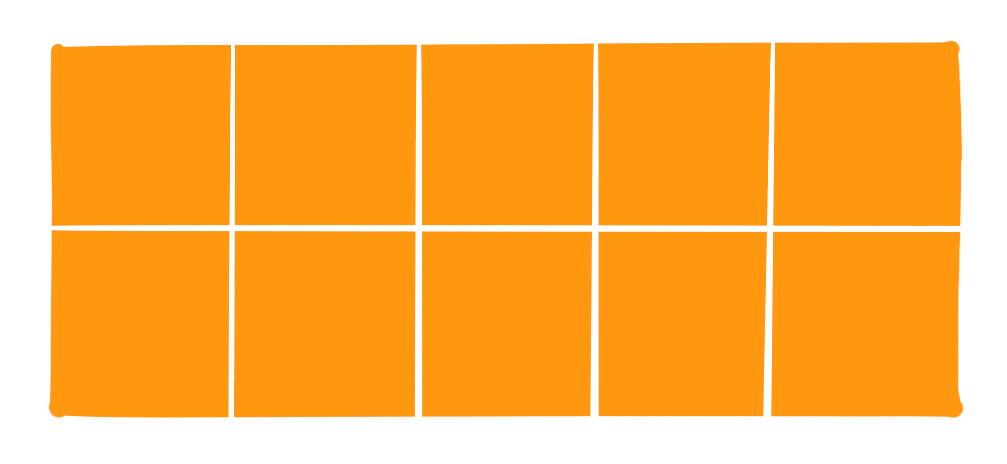
One spectacular example of this can be seen in the physical/digital system of “Multiplication by Heart” by Dan Finkel and Katherine Cook of Math for Love. Teachers oftentimes say that multiplication can be thought of as repeated addition:
They also say that multiplication can be thought of as finding an area:
Multiplication by Heart puts both of those together, so you’re constantly thinking through multiplication as both of these metaphors, and another one — the prime factorization system they created for their game Prime Climb.
How might we start small, now?
Watch my favorite CGP Grey video, “Rules for Rulers” — which does this for governing. Feel free to discuss it in the comments.
10. Related patterns
Like I said, this is implicit in all teaching — but an Egan education builds this into the scope and sequence of the whole curriculum, emphasizing diversity in part to help us see generalities.
In social studies, Customs and Cultures° makes anthropology a staple of middle school, in part to get a glimpse at human universals. In science, Biology of Imaginary Creatures° creates artificial diversity (“say you colonize a lifeless planet only with cats, sunflowers, and ants, and then wait 500 million years… what sorts of animals will you see?”) to plunk down faster into evolutionary processes. In math (as stated above), entering multiple solution methods into one’s Deep Practice Book° helps secure the most basic ideas.
And in language, Other Alphabets° and One Very Different Language° lead us to see what makes our alphabet and our language special.
Afterword:
In my review of The Educated Mind, I said that Egan’s quest could be called “getting to Plato” — helping everyone achieve the sort of powerful, beautiful, complex kinds of understandings that the world’s first great philosopher gelled together.
Well, in this pattern, I feel like we’re finally uncovering a big piece of how Plato’s mind worked. Dang, it’s fun! Thanks for reading and supporting this.





> Watch my favorite CGP Grey video, “Rules for Rulers” — which does this for governing.
Mine too! This was the first CGP Grey video my son sent me. It also has interesting implications for the “levers of power” that shape education policy.
For example, note which items in school we repeat the same way in order to help students conform (eg, pledge of allegiance, schedule bells) versus those we repeat in different ways to help them understand…