1. A problem
Brains weren’t built for schools. The K–12 academic curriculum is a foreign thing to evolution; many of the concepts we make students learn don’t easily fit inside human minds.
Some students struggle. Some never master the foundational concepts; for them, each new problem becomes multiple problems they must solve.
For them, a subject like math becomes harder and harder. For them, school becomes synonymous with failure.
2. Basic plan
For the big concepts in math — especially the ones that
will come up again in future years, and
are composed of finicky interlocking parts —
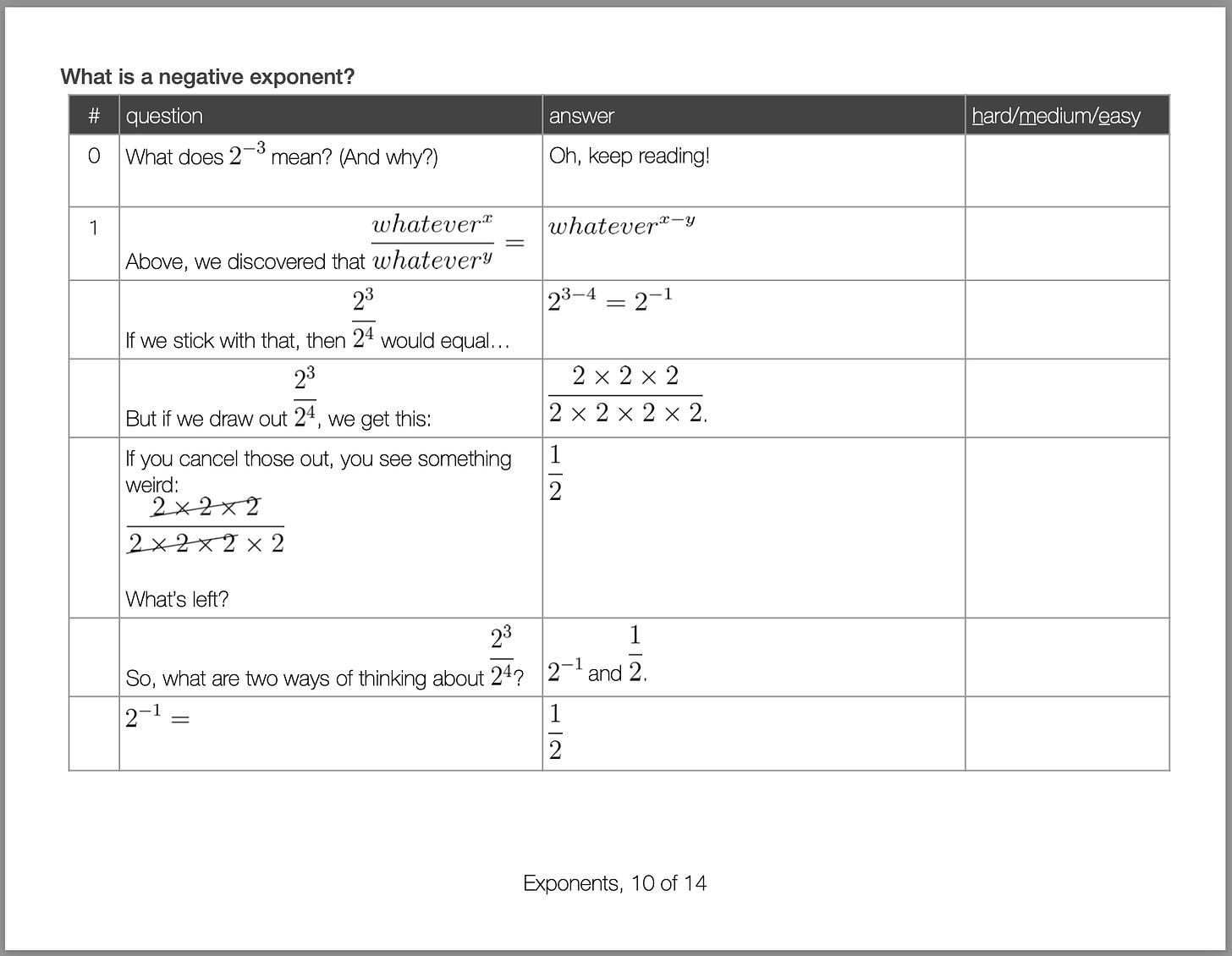
create two-column tables, with questions on the left, and answers on the right.
Each row gives a simple problem, so simple it can be done in a student’s head, with no need for pencil and paper. The problems on the first rows are below the student’s ability level — they’re what the student already knows.
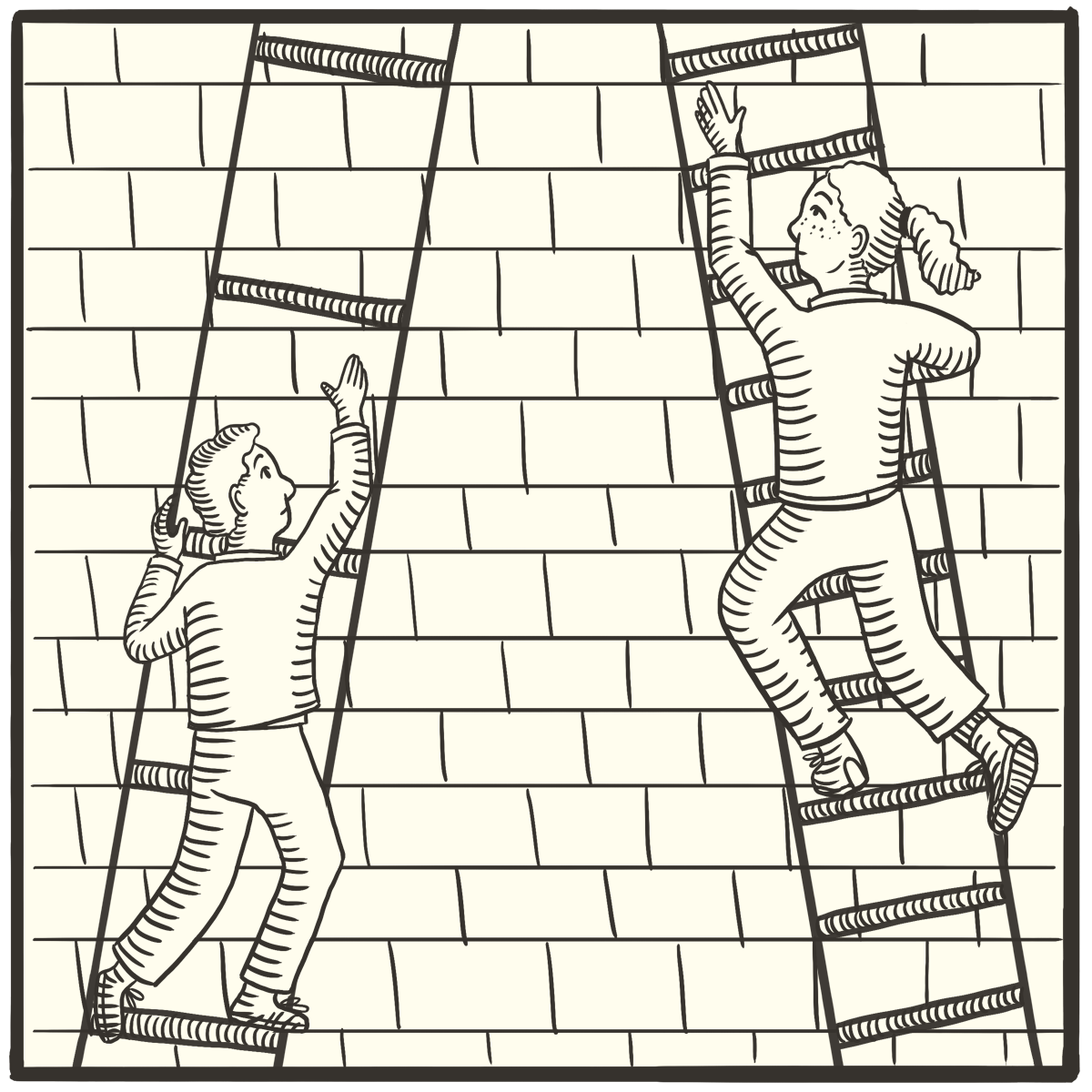
But then they build. Each problem goes a little beyond the last, never mindlessly repeating, but pointing out a new wrinkle, or exploring a new context.
Students “climb the ladder” by going through these either by themselves (covering up the “answer” column with a folded sheet of paper) or with a partner.
At the end of the table are problems that would have, just a few minutes before, seemed like sorcery:
And in an (optional) third column, they make note of whether they were able to get each answer — marking with a green ✅ or a red ❌. This helps them see precisely what they don’t understand — and gives them something specific to ask their teacher about. (They can even write down a question about that specific problem in the margin.)
Each day, they can re-attempt the problems they got wrong, until they’re understood. And each day, they can re-quiz the problems they had a hard time with (but now understand) until they’re automatic.
3. What you might see
Students going through Concept Ladders° when they didn’t understand something in math, or grammar, or punctuation, or really wonky science.
Students bringing these home as homework, and going through them verbally with a parent.
Students walking around with their chests out, proud that they’ve fully understood some hard crap.
Heck, you’re probably imagining more ways these could be used right now! Commenting on these weekly pattern language posts is a perk for paid subscribers, but you can become one for just $5/month.
4. Why?
Few students leave high school able to do high school math. (Or write a sentence without butchering standard English grammar.1)
This doesn’t need to be the case.
Math concepts (and grammar concepts, and science concepts) are analytical — they can be broken down into smaller pieces. Each piece can be understood by a normal human mind.
Students’ frustration with these subjects comes when we expect ‘em to…
hold multiple pieces in their heads at once, as a Educational Traditionalist might do in a lesson,2 or
construct these understandings themselves, as an Educational Progressivist might do in a lesson.3
Concept Ladders° are not the primary way we should be teaching math. But when it comes to the fundamental concepts — the ones which, until they’re automated, guarantee a world of pain — students deserve a “nuclear option”. They need a way to guarantee they understand the concept, and can keep it understood.
Concept Ladders° are that nuclear option.
5. Egan’s insight
Where do we see this in the human experience?
I used to imagine that the pedagogical technique of breaking things down into small steps, then repeating those steps again and again with care, was a demonic invention of the Industrial Revolution. More and more, though, I’m suspecting that it’s the way humans have used for millennia to learn hard skills.
Imagine a Hadza man learning to make arrowheads, or a !Kung San woman learning to identify medicinal plants, or an Aeta child learning to climb trees for honey.
We don’t need to apologize for repetition. It’s part of how all humans have gained talent.
How might this build different kinds of understanding?
Reminder: At the heart of Egan’s understanding of education is the notion that, for humans, certain practices and formats of information are special. Egan calls them “tools”, because throughout history, cultures have used them to pass themselves down. He groups them into five “toolkits” — SOMATIC (🤸♀️), MYTHIC (🧙♂️), ROMANTIC (🦹♂️), PHILOSOPHIC (👩🔬), and IRONIC (😏).
Well.
Doing the same thing again and again uses the natural human drive for 🤸♀️REPEATED ENGAGEMENT that we do even as babies. Breaking down a complex skill into 🧙♂️SIMPLE QUESTIONS leans on our natural facility with those. And the whole process emulates the engaged back-and-forth of 👩🔬SOCRATIC DIALOGUE that tries to find holes in a person’s reasoning, and quickly build new ideas.
6. This might be especially useful for…
Students who struggle to learn math (and other analytical concepts)
Students vary in their academic capacities — a fact which is, alas, sometimes objected to, but which might just be the single most well-attested fact in the hundred-fifty years of educational psychology!
This is great for them, because it lowers the cognitive load of a skill.
Every other student in the classroom, too
Every teacher faces a problem: what to do with stragglers? When a few students don’t understand something, how much focus should the teacher take from the class and give to helping them catch up?
When everyone in the class is able to follow along, every student benefits.
7. How could this go wrong?
Too effective; obviate the need to Eganize lessons
I made a mess of these when I was a tutor, and found that they were super-effective — so much so that I can imagine a teacher who has access to these might stop caring about Eganizing their lessons — bringing out what’s emotionally electric about the content.
That would be bad, as the goal of Egan education is more than just “help students get right answers”, but to help them experience what they’re learning as meaningful. (This, as I’ve observed, is the hole in the heart of education.)
Concept Ladders need to be a safety net, not the main teaching event.
Errors creep in, never get removed
Every piece of curriculum has mistakes, but a mistake in a Concept Ladder has the danger of making it into the heads of scores of students.
Perhaps we could issue rewards for students who find errors?
Can you think of another way this could go wrong… or, um, a way to help avoid that? Become a paid subscriber and join in the comments conversation!
8. Classroom setup
A question: should we make these available for students to access on their own? (If so, we need packets of each of these to be sitting on a shelf for students to access. If not, these can just be on a shared Google Drive, for teachers to print out as they need.)
9. Similar stuff (others are doing)
Direct Instruction (hereafter “DI”) is a hyper-traditionalist way of teaching; with it, teaching becomes the performance of literal scripts.
Almost everyone agrees on two facts:
It works, and it works especially for students who struggle with math.
Lots of teachers hate it.
Concept Ladders are a way of doing DI, minus the teacher. It’s a script written in question-and-answer form that the student can (usually) do by themselves.
10. Open questions
Does AI do this?
I’m entranced by the new generation of AI-powered math tutors, because they can break up a concept into small questions. If classroom math instruction is going the way of the dodo, maybe this isn’t necessary at all!
What else could this be used for?
As you’ve seen, I’ve made quite a few of these for math, and at least one for science. Grammar is another field that Concept Ladders° could be wonderful for… but what else?
11. How could this be done small, now?
I’ve created a bunch of these, and am happy to publicly share ‘em! Here are some for algebra, some for exponents, some for roots,4 and some for imaginary numbers.
I invite criticism in the comments! We’ll need people to make more of these — eventually, LOTS more of these. If anyone is interested in getting a start on this, lemme know. I’d be happy to provide feedback.
12. Related patterns
This is quite similar to Microscaffolded Math°, in which a big concept is broken into a bunch of small problems, which are so simple they can be given in a quick back-and-forth conversation with the class.
Identifying the specific moves that a student struggles is, implicitly, a way of Teaching Deliberate Practice°.
And locking them into long-term memory probably requires that we include these into students’ Spaced Repetition° collections.
Afterword: Going full-meta, here
Perhaps you’ve noticed that I haven’t posted much lately! “Alas,” you’ve perhaps said to yourself, “Brandon has grown lazy on his break. Ah, to be a teacher!”
Quite the opposite. I’ve been building up a backlog of these patterns, which have been scheduled twice a week from now until September. Look for ‘em on Wednesdays and Fridays.
“Bah!” you say, “Standard English grammar needs to be butchered!” Yeah, that’s an opinion. We’ll come back to that, by and by.
“All you need to do are these eighteen steps! Okay, now you try.”
“I know this took mathematicians hundreds of years to figure out, but here’s a colorful set of blocks, let me know if you need any help.”
Moving this one to Google Docs or whatever caused a couple image problems AND some of the end isn’t finished, but dang the rest of it is good; if you’ve always wanted to do cube roots in your head intuitively, take a look.






I really like this pattern! The main challenge I see with applying it is to break down a concept enough for the target audience to follow along. For example, the article that was shared about Squirrel AI says they've broken down the math curriculum into 10,000 separate steps. That's pretty intimidating for the average teacher to try to reproduce.
My current project is to try to help my two-year-old son learn to count. He loves to read his counting books, and we've read some of them literally hundreds of times. But so far he still seems to be missing the point. He thinks that counting means saying the words, "1, 2, 3", but he hasn't figured out that he's supposed to match the numbers with the objects that he's counting. (He also stops at 3 - anything larger than 3 is "so many").
After reading this post and thinking some more, I think maybe the concept of one-to-one correspondence is actually a prerequisite for counting. I have some ideas to try on how to make that more concrete for him, but I'd love to hear if anyone else has suggestions.
Lastly, I read the examples of concept ladders that you shared. Unfortunately, it's not quite true that sqrt(x^2) = x. That works for positive numbers, and maybe you're assuming the students haven't learned negative numbers yet? But in general, sqrt(x^2) actually equals the absolute value of x.
The mathematician's explanation would be that you can only invert a function that's one-to-one, but just typing that sentence makes me start to feel like this teacher:
https://www.smbc-comics.com/?id=3565#comic
Separate comment to address a general "how could this fail?" point that applies to a lot of things related to math. There's a general principle that students should learn not just the "how" but the "why", that they should understand rather than just learn a set of boring old rules. This is at least 95% true, but it has to be done in a way that avoids a trap.
Imagine you teach someone that 1 + 2 is the same as 2 + 1, and so on, so you can do a sum in any order you like (they're probably too young to need to know the difference between "associative" and "commutative"). Maybe later on they learn that 1 * 2 is the same as 2 * 1, and there's a general pattern here. The trap to avoid is that halfway through a longer problem, they encounter 1 - 2 and remember "you can swap things around in arithmetic" and turn it into 2 - 1.
Or you teach them how to cancel products in fractions (2*3/2*5 = 3/5) and next thing you know, they cancel (2+3)/(2+5) = 3/5 too. We've all been there. Lockhart's lament, which I unfortunately couldn't join the reading group for, is big on building mathematical intuition; there's some book-length arguments on similar topics by Jo Boaler that I'm familiar with too. The trap is that if student A is encouraged to use her intuition, and generally gets the right answer that way, that's good; if student B uses their intuition and ends up cancelling sums, that's not good. Similarly with Lockhart's "area of a triangle with height h and base b" example, if student B thinks about it and comes up with "h + b" as a result, that's wrong, even if they have a mathematical-ish justification for it: "well if you increase the height then the area gets bigger, and if you increase the base then the area also gets bigger, and both of those principles apply if you add the two". There's a danger that student B learns the meta-rule "they tell me all the time to THINK, but every time I do that I'm told I've got the wrong answer. Maybe it's just a lie they tell children." The basic risk to all attempts at building mathematical intuition - which is not just good, but necessary too - is that until it's fine-tuned enough in an individual student, it can produce random results and lower grades than just learning a formula. That doesn't mean one shouldn't do it, but that one actively has to watch out and intervene to help the "student B" types along the way.
I've privately tutored a few students in the past who were struggling with school math taught in a "learn to think" style, and sometimes they were so confused by the problems that they fell back on heuristics like "find all the numbers in the word problem and multiply them" because at least that looks like they're doing something to the teacher. They were a lot happier, and their grades improved, when for some categories of problem that made up a good part of their tests we went through explicit formulas or procedures that they could just reel off in the test without "thinking". Classic example: the math rule of 3 for proportions (2 apples cost $8, how much are 5 apples?). After seeing my students struggle with the "just think about it" approach, I taught them the old-fashioned method: make a 2x2 table, "scenarios" go in rows, label the columns ("amount", "price") then multiply the two things diagonally opposite and divide by the one in the corner. That completely hides why it works, but it got some of my students from a failing to a passing grade.
A related problem is that in math especially, I find it's often much easier to get an intuition for some rule in general, than it is to properly sort out the edge cases when it does or doesn't apply. This is as true for "higher" math like calculus (famous example: de l'Hopital's rule - a minefield of edge cases if there ever was one), and the example you presented here (the intuition looks like it should extend to 0^0, which I'm sure is covered in the full version of your notes), and for example it's easy to reason from 2x=2y giving x=y and 3x=3y giving x=y and so on that ax=ay always reduces to x=y. That doesn't mean you can't build this up as a concept ladder (in fact it's a great idea), as long as you make sure the student doesn't think they've understood before they get to the warning about if a could be 0.
I think my ideas here are best seen as a complement to, rather than a rebuttal of, concept ladders - which I really like as an idea by the way! Or perhaps being aware of this failure mode is a way to make much more effective concept ladders and intuition-building exercises.